Chapter 2
The Philosophy of Existence
2.1 The horizon of cognition
This is a philosophical explanation of contemporary scientific knowledge and mysteries that relates to the philosophical observations and theories published in EXISTENCE – a Philosophy for Life by Josef Zezulka1 [1] and in Spacetime + Gravity [2] by Tomáš Pfeiffer2, Josef Zezulka’s student and successor.
As we know from the theory of the general relativity [3] non-Euclidean geometry is of great help when describing the universe. In this view, space-time is curved and allows the existence of objects that can never be directly observed – singularities that curve space-time so much that not even light escapes them (black holes3). The theory of black holes defines an event horizon [21], beyond which no events could observed by an outer observer.
However, by philosophically observing the foundations of our world we can understand the curvature as a fundamental and integral part of the reality we live in.
Due to this curvature, our ability to observe is always limited by the “horizon of cognition” = the limiting boundary beyond which we cannot see (perceive, observe) anything.
This does not only relate to the material world (such as quantum mechanics and astronomy) but also to all non-material aspects such as psychology, philosophy, history, culture, and social, spiritual and health aspects or any other aspects – basically, anything that we can perceive, observe, assess or quantify in any other way.
Horizon of cognition is a general law that applies anytime, anywhere and everywhere.
From a scientific perspective we can relate this to the value of measurable intervals and how they correspond to the actual size in a curved space. The Fig. 2.1 illustrates the horizon of cognition in relation to micro and macro worlds [2]. The Figure highlights the meaning and importance of the axis’ intersection, which is the observer’s position4; i.e. the point where the interval begins.
As Spacetime + Gravity puts it [2], page 8:
„An interval can be understood as anything we can quantify – what we perceive, measure, evaluate, i.e. anything in our world. Therefore, it concerns mathematics, physics, astronomy, psychology, philosophy, etc. The following figure implies the importance of the axis origin – the point where the interval starts – the position of the observer. (...) When in proximity to the horizon of cognition, no matter how large the interval in the curved spacetime is, it no longer increases the interval on the fictitious linear axis. This is what I (Tomáš Pfeiffer) call the horizon of cognition beyond which it is not possible to observe, i.e. measure, anything anymore. It is always true for both directions – towards the universe and the atom, i.e. for light-years and angströms. (...) Nonlinearity is also manifested in the fact that any attempt at measurement is burdened by error being infinitely small at small intervals and, conversely, infinitely large at large ones.“ [2]
Our ability to observe the micro and macro world is thus essentially limited. So we are in fact observing (and hence also measuring and quantifying) the world in the plane of a right-angled Euclidean grid. But in reality, the world is curved in such a way that, once we reach the horizon of cognition, we lose the ability to measure, recognise or quantify anything at all.

Figure 2.1: Horizon of cognition [2] - schematic illustration. „This image illustrates the relationship between the value of the measurable interval and its real size in the curved space. This relationship is generally applicable to both macro and micro world. Around the horizon of cognition we are no longer able to determine the position as a change of the interval. There is an uncertainty when quantifying anything, both time and space.”
In our observation, our perception is always linear5.
Real space (Earth, the universe) is always curved6.
Therefore, there is a horizon of cognition, beyond which we are not able to observe anything.
And so when we look at the micro world at the horizon of cognition, the spacetime curvature makes the intervals “twist”, i.e. in our linear perception they shorten and form a line, one behind the other (their number grows infinitely) and we thus lose the ability to measure, quantify or differentiate between them in any way.
Analogically, the increments of the large intervals (which are also intervals), when looking at the macro world at the horizon of cognition, also “twist” due to the spacetime curvature, i.e. in our linear perception they shorten and form a line, one behind the other (their number grows infinitely) and we thus lose the ability to measure, quantify or differentiate between them in any way.
And so we can build ever bigger particle accelerators and we can construct ever bigger telescopes – and yet (due to the curvature) our ability to discover and observe will keep decreasing (as illustrated by Fig. 2.1, increasing the intervals on the x-axis will no longer improve our ability to observe the actual interval in a curved space).
Regarding our ability to observe and differentiate, there is no difference between observing the macro and micro world.
Contemporary physics measures and quantifies in metres, Joules and kilogrammes (physical intervals) – but also these are all merely linear ways of measuring intervals and depend solely on the observation point, on our position in relation to the object in question. The law of the horizon of cognition is therefore key if we want to understand the relation between the micro and macro world [2], page 15:
„The mathematics of large intervals very much differs from our linear mathematics. For instance, the lifetime of a particle measured in a particle accelerator, e.g. 10-5 s, de facto represents millions-of-years intervals within the particle’s spacetime, since the law of relativity (law of the horizon of cognition) applies to everything; The result of measurement is therefore not real but a fiction of a linear mapping (...). The same relations are also true in determining the particle energy and mass.” [2]
As already stated in Fig. 1.2, if we measure the dimensions of atoms in the order of magnitude from 10−11 to 10−10 m from our observation point, in the spacetime of the relevant atoms its dimensions may range from 1013 to 1014 m. While an atom in the micro world may have a rest mass of 10−27 to 10−26 kg from our observation point, an observer in the micro world would measure up to 1030 kg etc.
Philosophically, we can imagine the horizon of cognition as a perfect sphere with the observer placed in the middle. The observer is completely enclosed in the sphere and cannot observe or discover anything beyond the borders of the sphere. If the observer changes his position (he can move in space as well as change his size), the entire sphere moves with him.
From the perspective of relativity, we could also imagine that the observer does not move (the observer remains the same), but that is the world that changes size around him. The effect is the same. When standing on a boat on a river, it is the shore that moves. But if you are standing on the shore, it is the boat that moves.
The change in the position/size of the observed subject is illustrated in Fig. 2.2. This figure shows that, from the horizon of cognition point of view, the concepts “smaller” and “more distant” are, in fact, interchangeable, and that, in our observations, micro-world objects are just as distant as the whole universe.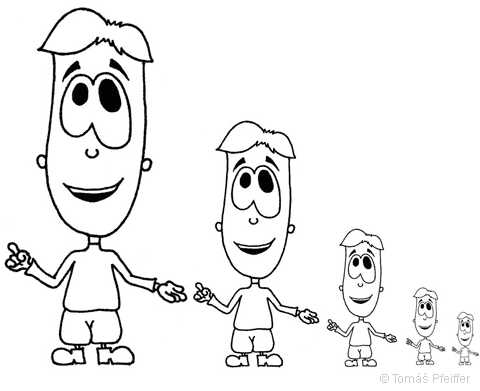
Figure 2.2: Changing position/size of the observer. Is the person on the picture getting smaller and smaller, or is he getting more and more distant?
1 Josef Zezulka was an eminent Czech healer and philosopher born 30 March 1912. As he turned 33 (30 March 1945) he had an extremely deep spiritual experience – an awakening. This moment changed his perception and his understanding of events and the world. His consciousness expanded. He received two gifts: the gift of healing and the gift of the spirit. With his gifts, he not only healed and founded the medical field Biotronics, he also started to teach people about the fundamental truths of life - the Philosophy of Existence. In time, his name became a concept that is known even abroad.
2 Tomáš Pfeiffer is Josef Zezulka’s successor. As Josef Zezulka’s student, Tomáš Pfeiffer heals and develops the field of Biotronics and also regularly lectures about and explains the Philosophy of Existence. He is the founder of the philosophical school The Spiritual University of Existence and of the Fellowship of Josef Zezulka – a new kind of religious society that aims to benefit of the entire biosphere. Please visit www.dub.cz/en/ for more information.
3 Black holes are objects with such a large mass (such a great gravitational force), that no object (including light) can leave its relevant spacetime region. The black holes were predicted by general relativity [3], and their existence is considered to be proven based on astronomical observations.
4 The term “observer” here refers to any form of live being, which is aware of its own existence, “its notion of existence - I am”. An observer may thus be also an animal, a plant, or any other possible life form.
5 5A linear dependence or relationship can be understood as a dependence, which can be mathematically described using linear functions (where there are, for instance, no powers etc.). The measured/observed output value is directly proportional the input value. A linear function can thus be expressed as: f(x) = ax + b, where a, b are arbitrary constants.
We can for instance imagine, that the longer my route is, the more time I need to spend on it (if my speed is constant). A relation between (x) and f(x) can also be considered as a relation between a cause and a consequence; then a small change to (x) causes a small change to the consequence f(x). A geometrical expression of a linear quantity is a straight line. If we measure the consequence f(x), such as the amount of time spent on a journey, an independent observer can provide exact information about (x) – the length of the route.
6 Non-linear dependence (showing curvature) is a dependence which violates the rules of a linear dependence. The relation between the measured/observed output interval f(x) related to the input interval (x) cannot be expressed by a direct proportionality. Let us imagine, that in one location, on an infinite, and ideally straight, highway there are two people, A and B. Then B starts to run, moving away from A on this very long, straight highway. A will easily be able to determine B’s starting velocity, and will still be able to see B getting smaller and smaller as the distance increases. However, the further B gets along the highway, the more tired he will become. Therefore, he will be gradually slowing down. The time spent on this journey will thus grow faster and faster as the length of the road increases. For A, who only has a linear measuring axis, it will become increasingly difficult to determine how far B has run based on merely the time B has spent on the journey (the error of its determination will grow). Should B, feeling increasingly tired, almost stop or take breaks, it becomes very difficult or almost impossible for A to exactly determine the actual distance B has run based purely on the time measurement. If A assumes that B is still moving away with at the same speed (as at the beginning, i.e. if A’s observation/consideration follows Euclidean axes), his findings (observations) will be burdened by a larger and larger error that increases along with the distance (interval) B covers.

